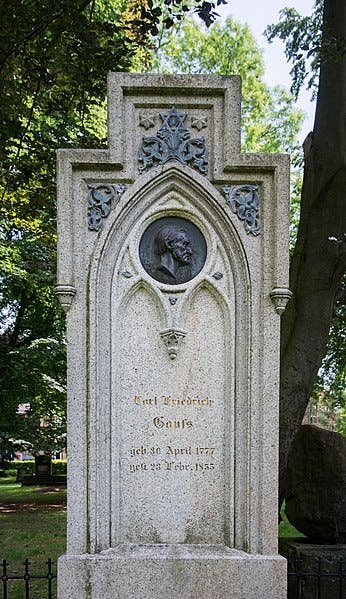Scientist of the Day - Carl Friedrich Gauss

Carl Friedrich Gauss, a German mathematician, was born Apr. 30, 1777.  It is hard to talk about mathematicians in these notices, because for those of us (most of us) who are not advanced mathematicians, it is difficult to appreciate their accomplishments, and simply saying that someone invented the method of least squares (as Gauss did) means little if you have never used the method of least squares. But this much we can all appreciate: in the estimation of many mathematicians and historians of mathematics, Gauss is considered one of the three greatest mathematicians in the entire history of Western civilization, which puts him in pretty good company, since his two companions in this triumvirate are usually Archimedes and Isaac Newton.

Gauss’s special interest was arithmetic, and his Disquisitiones arithmeticae, published in 1801, demonstrated to all contemporary mathematicians that a genius walked among them. Gauss finished the manuscript in 1798, when he was just 21 years old, so one might guess that Gauss was a child prodigy, which indeed he was. He had a photographic memory, as well as an uncanny ability to do long calculations in his head. It was said that when doing astronomical calculations that required logarithms, which are typically 10 digit numbers in log tables, he would often just recall the logarithms instead of bothering to look them up.

“The discovery of Ceres,” headpiece from Giuseppe Piazzi, Della scoperta del nuovo pianeta Cerere, 1802 (Linda Hall Library)

Although he started out as a pure mathematician, Gauss ventured into astronomy in 1801, when the first asteroid, Ceres, was discovered (second image). The discoverer, Giuseppe Piazzi, observed Ceres for only a short while before it was lost behind the sun, and he did not have enough data to calculate its orbit and thus predict where it might be at some future time. Ceres had been discovered and then irretrievably lost, or so it was thought.  Gauss saw this as a suitable challenge for a mathematician, and in reasonably short order, he worked out a method to generate the asteroid's orbit from the few observations gathered by Piazzi. Sure enough, Ceres was spotted later that same year, reappearing on the other side of the sun at the very position that Gauss predicted. Gauss published an account of his new methods of calculating orbits in Theoria motus corporum coelestium (1809), and these techniques were to become essential equipment for later astronomers, as more and more asteroids were discovered and lost in the century to come. We have both the Disquisitiones arithmeticae and the Theoria motus in our History of Science Collection, along with many other works by Gauss.

Statue of Gauss, Braunschweig, Germany (Wikimedia commons)

Modern mathematicians have recently begun composing and collecting one-liners involving Gauss, most of them making the same point, that Gauss had mathematical powers denied to the rest of us. Two examples are “Gauss can recite the digits of π – backwards", and “Gauss played himself in a zero-sum game, and won $50." There is at least one website devoted to these Gauss jokes, where they are called "Gauss facts”, which seems to be a lame name for a joke genre.  We would prefer “Gaussism” and hereby coin the term. If you scroll through this website, where there are many pages of Gaussisms, you will see why few mathematicians are hired as writers for the Jimmy Fallon Show. But there are a few, such as the two we chose above, that are worthy of the great Gauss.

There is a statue of Gauss in Braunschweig in Lower Saxony (third image), and a bust on his funeral monument in Göttingen (fourth image).  The oil portrait is in the collection of the Berlin-Brandenberg Academy of Sciences and Humanities (first image).

Dr. William B. Ashworth, Jr., Consultant for the History of Science, Linda Hall Library and Associate Professor, Department of History, University of Missouri-Kansas City. Comments or corrections are welcome; please direct to ashworthw@umkc.edu.