Scientist of the Day - Gottfried Wilhelm von Leibniz
Gottfried Wilhelm (von) Leibniz was born on July 1, 1646 in Leipzig, Germany, and was baptized into the Lutheran Church three days later. Leibniz’ father, a philosophy professor at the University of Leipzig, died when the boy was only seven, leaving him to be raised by his mother Catherina. From that moment on, Leibniz had access to his father’s splendid library of both classical and modern works, most written in Latin and Greek, which the young man soon mastered. At age 15 he entered Leipzig University, where he got his bachelor’s degree in eighteen months, and, in rapid succession, completed a master’s in philosophy, a bachelor’s in law, got his habilitation in philosophy, and, in early 1666 wrote his first book De Arte Combinatoria, which was to introduce topics that would interest – indeed, entrance – him for the rest of his life.
Leibniz made contributions to many fields – mathematics, logic, physics, geology, law, mining, diplomacy, theology, philosophy – he was one of the great polymaths of all time. His contributions to mathematics, logic, and what today we call computer science will concern us today. In mathematics, it is well known that Leibniz and Newton independently discovered (? Or was it “invented”?) the calculus, both differential and integral. At the time, Newton tried to grab all the glory for the invention for himself, claiming – much to Leibniz’ surprise – that Leibniz had stolen the ideas from him. Newton’s animosity toward Leibniz continued until the latter’s death in 1716, resulting in Leibniz being left behind when his employer came to England to be the next king. Newton also engaged Leibniz by proxy in a final dispute over the nature of space and time during the correspondence between Leibniz and Samuel Clarke, who was Newton’s mouthpiece during the interaction.
Yet Leibniz’s mathematical interests ranged far beyond his controversies with Newton. Early in his development, he had discovered the system of binary numbers – “rechnung mit null und eins” (“calculating with 0 and 1”) – and systematically worked out the arithmetic and algebra of the dyadic system. He soon saw deeper meanings and connections between his developing binary system and the wider universe. In metaphysics, for example, he thought that God would be the exemplar of oneness, unity, while the void would be zero; together the universe and all in it would be the result of the interaction between the oneness of God and the zero of the void.
Another long-term project also tied into Leibniz’ binary number system: his desire to find unity among all religions and theologies. To this end, he had come into close contact with the Jesuit Joachim Bouvet, who was engaged in missionary work in China. Bouvet satisfied Leibniz’s burgeoning interest in Chinese language, culture, and, especially, philosophy, with a constant stream of literature and artifacts. One of them, a diagram of I ching hexagrams, especially caught Leibniz’s attention. He soon realized that the diagram’s figures encoded a straightforward counting system expressing the binary numbers! This discovery, Leibniz believed, provided deep evidence of his conviction that all religions shared a fundamental basis—Christian, of course!
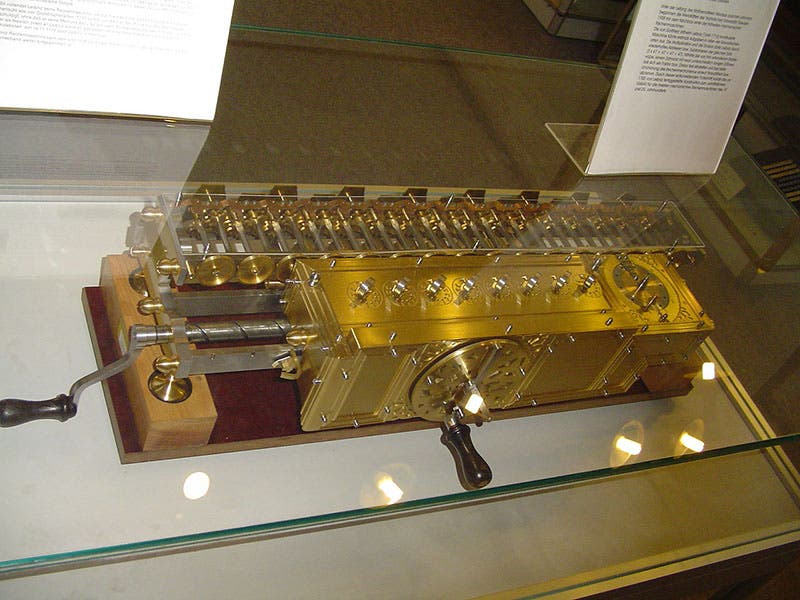
In 1670, Leibniz built a mechanical calculator that could do all four arithmetic operations. Such a machine wasn’t unknown – the French mathematician Blaise Pascal had built one in the 1640s to help his father, who was a government tax agent, compute tax forms, and he had perfected the design throughout the next several decades. In 1672, Leibniz began a several-year stay in Paris, where he had access to Pascal’s work, including the final model of his calculator. Leibniz continued to work on his own machine, and the following year took it with him to London, where he made a presentation to the Royal Society. Its members were so impressed with both the man and his machine that they made Leibniz a fellow of the prestigious society.
Leibniz continued to work on his machine over the years, famously carrying it around Europe with him, taking it to established artisans and craftsmen in the various cities he visited. A major problem facing him – and anyone trying to build a mechanical calculator – was mechanizing the carrying function, the process of going from one vertical column to the next, e.g., in the decimal system, of going from the 10’s column to the 100’s column when you add 1 to 99. Leibniz solved the problem finally and elegantly in 1694 with the step wheel, which is now called “the Leibniz wheel.” Leibniz’s solution was so perfect that it was used until the advent of the electronic calculator in the 1970s.
Once he had perfected the carrying function, Leibniz was now able to produce a complete, smoothly functioning mechanical calculator, which he promptly did. We see here a replica in the Technische Sammlunger Museum in Dresden (second image). In effect, Leibniz produced the first modern mechanical calculator.
We are left with an intriguing puzzle: Did Leibniz ever see the connection between his binary system and his calculator? After all, his calculator was digital, it worked on the base ten. But the binary system is base two. Leibniz knew full well that the binary system was not only complete, and was, in certain ways, more effective and efficient than the decimal system; did he ever see that it might have a role to play in mechanical computation?
This question remained asked but unanswered until very recently, when a pair of German researchers, digging deep into Leibniz’ unpublished papers, found exactly what they needed to answer the question. Here is a figure from their paper that shows Leibniz’s notes of 1679 and a transcription. In his unpublished paper, Leibniz summarized how the four basic arithmetical operations would be done in binary and how conversion between digital and binary could be carried out. After laying out the theory, Leibniz immediately entered into a discussion of how the binary arithmetical operations might be mechanized: “This type of calculation could also be performed with a machine (without wheels) … Using a box with holes that can be opened and closed.” What Leibniz has in mind is a box with moveable slots, holes, and a device that can release no ball, one ball, or one ball plus one ball, in a sequence of movements. By a complex series of back and forth movements, the basic four operations could be carried out. Although Leibniz himself never built the machine, his design directions are clear enough that researchers at the Deutsches Museum in München built a working model of such a machine in the early 2000s that worked perfectly.
Leibniz truly was a universal genius. But what is amazing is that his work so long ago – he died over 300 years ago! – is still relevant today.
George Gale is Professor Emeritus of Philosophy, University of Missouri – Kanas City, and the author of Dying on the Vine: How Phylloxera changed the World of Wine; comments are welcome: galeg@ukmkc.edu.