Scientist of the Day - Benoit Mandelbrot
Benoit Mandelbrot, a Polish/French/American mathematician, was born on Nov. 20, 1924. He and his family fled Poland for Paris when he was 12, where his latent mathematical abilities came to light. He was admitted to the prestigious École normale supérieure when he was 19, but almost immediately, he dropped out and entered the École polytechnique, because he was more interested in the applications of mathematics to the real world than he was in theory. This concern would mark his entire career. He accepted a summer job at the Thomas J. Watson Research Center in New York City, run by IBM, in 1958, and would end up staying there for 35 years before finally moving on to a professorship at Yale.
Benoit Mandelbrot, photograph (The Times)
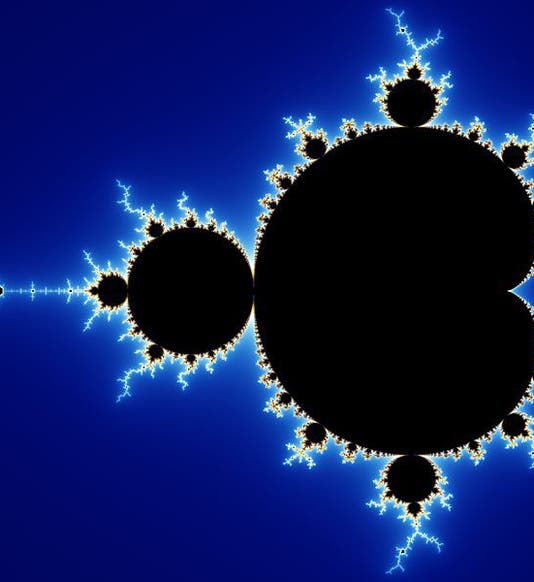
Mandelbrot was interested in power laws, which compare the frequency of events to the sizes of those events. For example, it happens that the most frequently used word in the English language is used twice as much as the second most frequent, and three times as much as the third most frequent, and so forth; if one graphs such a law, the curve is high at the left, drops rather swiftly as it moves to the right, and then tapers off slowly. Many kinds of events obey power laws: the frequency of family names, earthquake intensities, commodities prices, the distribution of wealth in society. Interest in power laws led Mandelbrot in the 1970s to the study of fractals, a term he coined in 1975 for an already existing geometry. Fractals are curves that can be generated by the simplest of algorithms, but have almost infinite complexity. The most interesting property of a fractal is what is called "self-similarity;" the perplexing fact that no matter how much you change the scale, by zooming in or moving out, it still looks the same.
Frost patterns with fractal properties (Wikimedia Commons)
For the non-mathematician, Mandelbrot's most important contribution was in demonstrating that fractal geometry, not Euclidean geometry, is the language of nature. Coastlines, mountain ranges, cloud patterns, tree growth--all these are better represented by fractals than any other means. Indeed, film animators now regularly use fractals to generate background details that used to be impossible by traditional methods. The most famous fractal is probably the "Mandelbrot set,” which inevitably appears whenever there is a discussion of fractals, complexity, or chaos theory (first image). You can zoom in on a Mandelbrot set with this animation. If you like your animations with music and are partial to Jonathan Coulton, try this one.
Coastline of Lake Nasser, Egypt, from Google Earth (paulbourke.net)
One aspect of Mandelbrot that we find compelling is that he had a great admiration for Johannes Kepler, the seventeenth-century astronomer, even calling him a role model. He liked the fact that ellipses had been around for two thousand years, with no practical application whatsoever, before Kepler gave ellipses meaning, by showing that the planets move in elliptical orbits. Mandelbrot said that he wanted to be like Kepler - to find a degree of order in some area where everyone else saw a "lawless mess." "My dream was to become the Kepler of complexity," Mandelbrot confessed in an interview. It would seem that his dream was realized.
The last two photographs above depict frost patterns on a pane of glass and a satellite view of the coastline of Lake Nasser in Egypt; both exhibit fractal characteristics.
Dr. William B. Ashworth, Jr., Consultant for the History of Science, Linda Hall Library and Associate Professor, Department of History, University of Missouri-Kansas City. Comments or corrections are welcome; please direct to ashworthw@umkc.edu.
